Around 2,000 years ago, the Greek astronomer Hipparchus set out to map the night sky and measure the distances between stars in the celestial sphere. His efforts produced a “table of chords,” long regarded as the oldest known table of trigonometry. For centuries, Hipparchus was celebrated as the 'father of trigonometry.' However, researchers from the University of New South Wales (UNSW) recently discovered that the Babylonians had beaten the Greeks to this mathematical breakthrough. After studying a 3,700-year-old Babylonian clay tablet, they found what is now considered the “oldest and most accurate trigonometric table.” The study was published in Historia Mathematica.

According to lead researchers Dr. Daniel Mansfield and Norman Wildberger, the Babylonian tablet was used by ancient scribes to calculate how to construct palaces, temples, and canals, as reported by Phys.org. Mansfield first encountered the tablet, known as “Plimpton 322,” while preparing material for first-year mathematics students at UNSW. He and Wildberger decided to explore Babylonian mathematics further and investigate the tablet's contents and significance. They soon realized it was connected to trigonometry. "Plimpton 322 predates Hipparchus by more than 1,000 years," said Wildberger. "The mathematical world is only now waking up to how much this ancient and highly sophisticated mathematical culture has to teach us."
Trigonometry is a blend of three words – tri (three) + gono (sides) + metron (measure), hence it deals with the relationship between sides of a triangle. This tablet depicts a system of 15 rows and four columns to describe a sequence of 15 right-angle triangles, which decrease in inclination as the triangle is squeezed downwards. Researchers reported that originally, the tablet had 38 rows, but they could study only 15 because the left edge of the tablet was broken.
All the text on the tablet was found to be written in the cuneiform script. "Plimpton 322 contains a special pattern of numbers called Pythagorean triples," said Mansfield. According to Wolfram Mathworld, a Pythagorean triplet is a triple of positive integers a, b, and c such that a right triangle exists with legs a, b, and hypotenuse c. The triplets form an equation in which the square of two sides is equal to the square of the hypotenuse: a² + b² = c². An example is integers 3, 4, and 5, which form the equation a²+b²=c². However, the values on Plimpton 322 were found to be considerably larger like a row representing the numbers: 119, 120, and 169.

"Our research reveals that Plimpton 322 describes the shapes of right-angle triangles using a novel kind of trigonometry based on ratios, not angles and circles. It is a fascinating mathematical work that demonstrates undoubted genius,” wrote the researchers.
Plimpton 322 was first discovered in the 1900s in present-day southern Iraq by archaeologist Edgar Banks. It was buried in the ancient Sumerian city of Larsa and now rests in the Rare Book and Manuscript Library at Columbia University in New York. "This is a rare example of the ancient world teaching us something new," the researchers said.
This article originally appeared last year.
















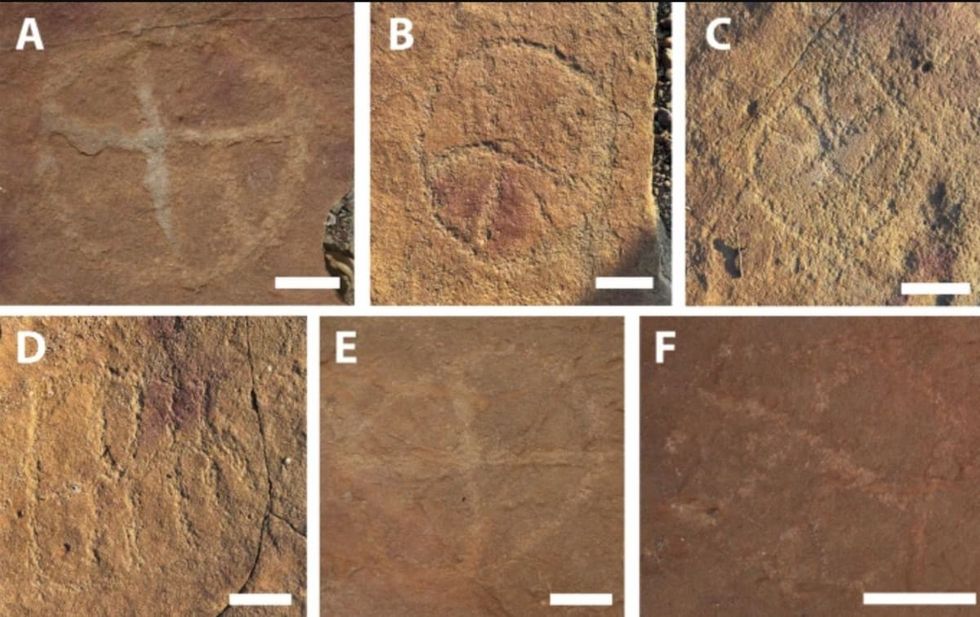 Image frmo Scientific Reports of ancient artwork. Image Source:
Image frmo Scientific Reports of ancient artwork. Image Source: 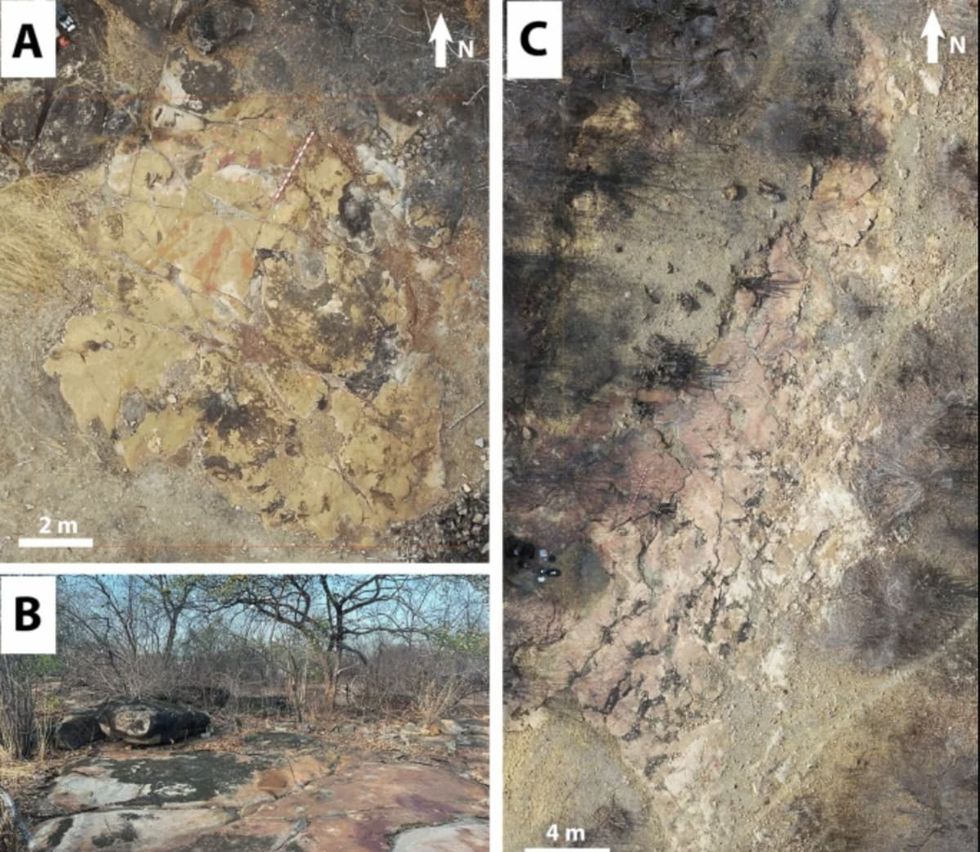 Image frmo Scientific Reports of ancient artwork.Image Source:
Image frmo Scientific Reports of ancient artwork.Image Source: 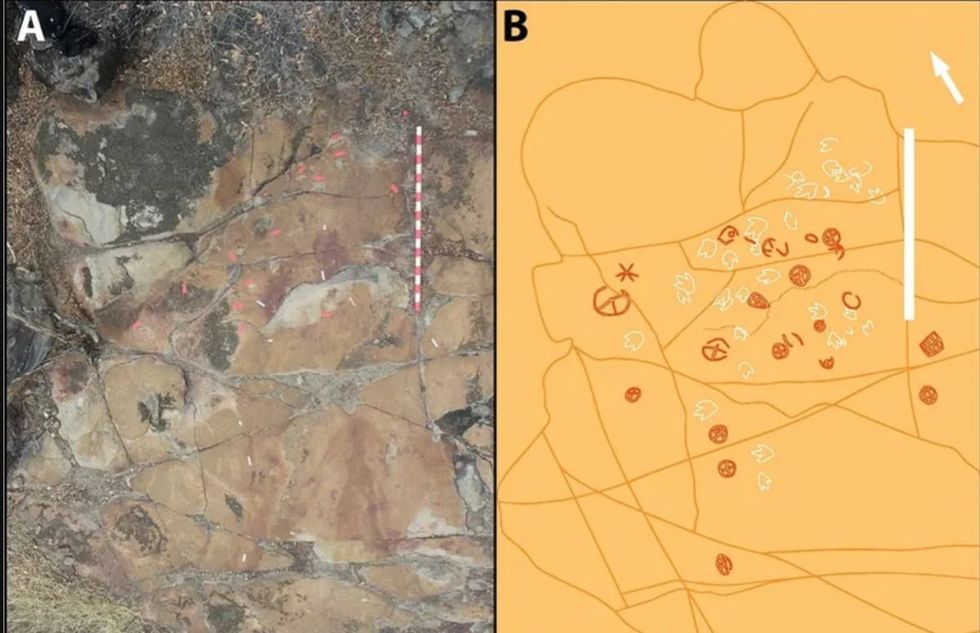 Image frmo Scientific Reports of ancient artwork.Image Source:
Image frmo Scientific Reports of ancient artwork.Image Source: 

 It's difficult to imagine seeing a color and not having the word for it. Canva
It's difficult to imagine seeing a color and not having the word for it. Canva
 Sergei Krikalev in space.
Sergei Krikalev in space. 


 The team also crafted their canoe using ancient methods and Stone Age-style tools. National Museum of Nature and Science, Tokyo
The team also crafted their canoe using ancient methods and Stone Age-style tools. National Museum of Nature and Science, Tokyo The cedar dugout canoe crafted by the scientist team. National Museum of Nature and Science, Tokyo
The cedar dugout canoe crafted by the scientist team. National Museum of Nature and Science, Tokyo